MOMENTUM AND IMPULSE QUESTIONS AND ANSWERS
Share via Whatsapp Join our WhatsApp Group Join our Telegram GroupWorked example 1
- A car has a momentum of 20 000 kg·m·s–1. What will the car’s new momentum be if its mass is doubled (by adding more passengers and a greater load) and it travels at the same velocity?
- What will the velocity be if the momentum is 60 000 kg·m·s–1 and the mass of the car is 2 000 kg?
- A truck has a mass of 6 000 kg and travels at 80 km·h–1. How does the momentum change if the truck is loaded with 1 200 kg and then travels at 60 km·h–1?
Solutions
- The formula for momentum is p = mv , so the momentum will double and will be equal to 40 000 kg·m·s–1 in the same direction as before.
- p = mv
60 000 = 2 000 × v
∴ v = 30 m·s–1 in the same direction as the momentum. - Convert both velocities to m·s–1:
vi = 22,22 m·s–1 & vf = 16,67 m·s–1
pi = mivi = (6000 × 22,22) = 133 320 kg·m·s–1
pf = mf vf = (7200 × 16,67) = 120 024 kg·m·s–1
Δ p = pf – pI = 133 320 – 120 024 = 13296 kg·m·s–1
Worked example 2
Why is it less painful for a high jumper to land on foam-rubber carpet than on the ground?
Solution
Fnet = Δp
Δt
The Fnet needed to bring the jumper to rest (vf = 0 m·s−1) depends on Δp and Δt. When he lands on the foam-rubber, he comes to rest over a longer period of time (Δt) than if he lands on the ground.
- So time taken Δt to change his momentum increases
- Fnet decreases (Fnet ∝ t )
Δ1 - The magnitude of Fnet determines the amount of pain experienced, so it is less painful to land on foam-rubber.
Worked example 3
- A spaceship has a mass of 1 000 kg. The rocket engines discharge for 5 s and increase the rocket’s velocity from 25 to 30 m·s–1. Calculate the force exerted by the engines to cause this change in momentum.
- Assume the direction of the initial velocity is positive and the answer you obtain in the above problem is negative, what would be the direction of the exerted force?
Solutions
- Let the direction of the initial velocity be positive.
Fnet = Δp = mvf – mvi = (1000)(30) – (1000)(25)
Δt Δt 5
= 1 000 N in the initial direction of motion. - The same i.e. in the initial direction of motion.
Activity 1
Study the diagrams below showing the movement of a 150 g baseball thrown at a wall at right angles.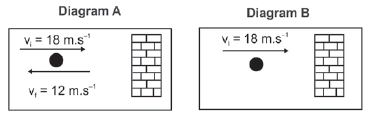
Diagram A:
m = 150 ÷ 1000 = 0,15 kg
vi = + 18 m·s−1
vf = −12 m·s−1
Diagram B:
m = 0,15 kg
vi = + 18 m·s−1
vf = 0 m·s−1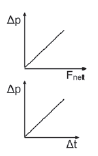
Let the direction towards the wall be positive.
- Calculate the momentum of the baseball as it strikes the wall in Diagram A. (3)
- Calculate the momentum of the baseball as it leaves the wall in Diagram A. (3)
- Calculate the change in momentum during the collision in Diagram A. (3)
- Calculate the force exerted by the wall on the baseball in Diagram A and in Diagram B, if each collision lasts 0,1 s. (6)
- Draw a vector diagram to illustrate the relationship between the initial momentum (pi), the final momentum (pf) and the change in momentum (Δp = pf – pi) for the baseball in Diagram A. (6)
[21]
Convert mass to kg!
Solutions
|
Activity 2
A cricket ball of mass 175 g is thrown horizontally towards a player at 12 m·s−1. It is hit back in the opposite direction with a velocity of 30 m·s−1.
The ball is in contact with the bat for a period of 0,05 s. Calculate:
- The impulse of the ball. (4)
- The force exerted on the ball by the bat. (3)
- The force exerted on the bat by the ball. Motivate your answer by referring to a Law of Motion. (5)
[12]
Solutions
|
Problem Type 1: Two objects collide and continue to move as separate objects after the collision
Activity 3
In a railway shunting yard, a locomotive (train engine) of mass 4 000 kg, travels due east at a velocity of 1,5 m·s−1. The train driver tries to link it to a stationary wagon of mass 3 000 kg by letting them collide. Instead, the wagon moves due east with a velocity of 2,8 m·s−1.
Calculate the magnitude and direction of the velocity of the locomotive immediately after the collision. (11)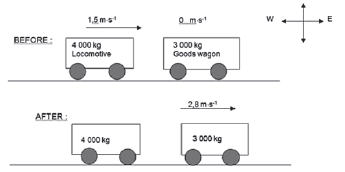
| Solution Let the locomotive be object 1 and the wagon be object 2. Let motion to the east be positive Then: m1 = 4 000 kg v1i = +1,5 m·s−1 v1f = ? and m2 = 3 000 kg v2i = 0 m·s−1 v2f = + 2,8 m·s−1 Σpi = Σpf m1v1i + m2v2i = m1vf + m2v2f (4000)(1,5) + (3000)(0) = (4000)v1f + (3000)(2,8) 4000 v1f = 6000 – 8400 v1f = 0,6 m·s−1 ∴ 0,6 m·s–1 to the west [11] |
Remember: If an object is stationary, its velocity is 0 m·s-1; "stationary" means "not moving".
Problem Type 2: Two objects collide and unite
Activity 4
A boy of mass 40 kg runs at 5 m·s–1 east and jumps onto a skateboard of mass 2 kg moving at 3 m·s–1 east.
Calculate the speed at which the boy and skateboard move together. (7)
| Solution Let the boy be object 1 and the skateboard be object 2. Let motion to the east be positive. Then: m1 = 40 kg and m2 = 2 kg v1i = 5 m·s–1 v2i = 3 m·s–1 v(1 + 2)f = ? Σpi = Σpf (40)(5) + (2)(3) = (40 + 2)vf 42vf = 206 vf = 4,9 m·s–1 3 ∴ the boy and skateboard move together at 4,9 m·s–1 [7] |
Problem Type 3: Two moving objects that are initially joined, then separate
Activity 5
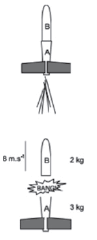
Hendrik is an amateur rocket builder. He launches a two-stage rocket as shown in the diagram. Section A (stage 1) contains the rocket engine and fuel. Section B (stage 2) has a mass of 2 kg.
- Hendrik says that Newton’s Third Law of Motion is used to explain why the rocket moves upwards during flight. Identify one actionreaction pair of forces involved with the rocket’s motion. (1)
At a certain height, when the rocket has a velocity of 5 m·s–1 upwards, the last fuel is used up, and section A has a mass of 3 kg.
To get section B even higher, a small explosion separates section B from section A at this point and increases the upwards velocity of section B to 8 m·s–1. - State the Law of Conservation of Linear Momentum in words. (4)
- Calculate the velocity of section A after the explosion. (11)

[16]
Remember: The question asks for the speed of the skateboard. Speed is a scalar, so you don’t need the direction of the motion in the answer.
Solutions
|
Problem Type 4: Two stationary objects that are initially joined, and then separated (e.g. during an explosion)
When two objects are forced apart by an explosion or as a result of a compressed spiral spring released between them, they move in opposite directions after the explosion e.g. when a gun fires a bullet, the bullet moves forwards and the gun moves backwards.
Activity 6
A gun of mass 1 kg is attached to a trolley of mass 4 kg and is loaded with a bullet of mass 2 g. The system is at rest on a frictionless horizontal surface. The gun is fired by remote control and the bullet has a muzzle velocity of 350 m·s−1.
Calculate the velocity of the trolley and gun after the bullet has been fired. (8)
| Solution Let the direction of the bullet’s motion be positive. Let the trolley and gun be object 1: m1 = 1 + 4 = 5 kg v1f = ? Let the bullet be object 2: m2 = 2g = 0,002 kg v1f = 350 m·s−1 Before the explosion: v(1+2)i = 0 m·s−1 Σpi = Σpf (m1 + m2)vi = m1v1f + m2v2f 0 = m1v1f + b2v2f m1v1f = – m2v2f (5)v1f = –(0,002)(350) v1f = –(0,002)(350) = –0,14 m·s–1 5 Therefore the gun and trolley move at 0,14 m·s−1 in the direction opposite to that of the bullet, after the explosion. [8] |
Problem Type 5: An object falls vertically onto another object that is moving horizontally below it
Activity 7
A trolley of mass 3 kg moves at 4 m·s−1, west along a frictionless horizontal path. A brick of mass 1 kg drops vertically onto it. The brick lands on the trolley at a vertical velocity of 0,5 m·s−1. Calculate the velocity of the brick and trolley system after the collision. [13]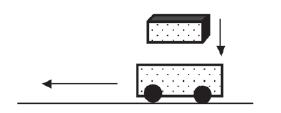
| Solution The brick strikes the trolley vertically at 0,5 m·s−1. So the brick’s horizontal velocity is zero (0 m·s–1). Momentum is conserved in a straight line. So the brick’s vertical velocity is ignored when applying the Law of Conservation of Linear Momentum. (3) Let motion west be positive Let the trolley be object 1: m1 = 3 kg v1i = +4 m·s–1 Let the brick be object 2: m2 = 1 kg v2i = 0 m·s−1 After the collision: v1 + v2 = ? Σpi = Σpf m1v1i + m2v2i = (m1 + m2)vf (3)(4) + (1)(0) = (3 + 1)vf Zero, because the brick is not moving horizontally 12 = (4)vf vf = 3 m·s–1 ∴ brick and trolley system have a velocity of m·s−1 west (horizontally) (10) [13] |
Activity 8
Collisions between vehicles take place on the roads in our country daily. In one of these collisions, a car of mass 1 600 kg, travelling at a speed of 30 m·s–1 to the left, collides head-on with a minibus of mass 3 000 kg, travelling at 20 m·s–1 to the right. The two vehicles move together as a unit in a straight line after the collision.
1. Calculate the velocity of the two vehicles after the collision. (8)
2. Do the necessary calculations to show that the collision was inelastic. (13)
3. New cars have a crumple zone to help minimise injuries during accidents. Air bags and padded interiors can also help to reduce the chance of death or serious injury. Use principles in Physics to explain how crumple zones and air bags can reduce the chance of death or injury. (9)
[30]
Solutions
|
Activity 9
A bullet of mass 10 g, moving at a velocity of 300 m·s–1, strikes a wooden block of mass 1,99 kg resting on a flat horizontal surface as shown in the diagram below. The bullet becomes embedded in the block. Ignore the effects of air friction.
- Write down in words the principle of conservation of linear momentum. (2)
- Calculate the speed of the block-bullet system immediately after the collision. (4)
- Is this collision elastic or inelastic? Give a reason for the answer. (2)
The floor exerts a constant frictional force of 8 N on the block-bullet system as it comes to rest. - Calculate the distance that the block-bullet system moves after the collision. (5)
[13]
Solutions
|